Relativity predicts a variable G
In the field of gravitation, current observations can indeed be explained by relativity — but only if relativity is extended with a new chapter that has so far been overlooked. This new development reveals that relativity predicts a variable gravitational constant, G. It is primarily this variation that resolves many of the outstanding mysteries in gravitational physics today. A complete demonstration of this new prediction is presented below.
(The above version is the latest; an archive version might be older). The proof starts by considering a dimensionless particle in an empty universe. Then two particles, three particles, and an infinite set of particles are studied. This allows to calculate space-time structure for any realistic energy distribution. The proof uses the interchange of limits theorem, and ad hoc sequences of energy distributions. With only one particle the result is a singularity everywhere if the universe is empty outside of the particle. Those singularities disappear completely with three particles. Then this calculation is done for any realistic energy distribution. An equation of G is given naturally in the process. This equation is a correct approximation in most of the cases. The fundamental principles building Einstein equation are still valid, but now the constant anthropocentric solar system value is shown to be weaker in strong matter density environments, and greater in low matter density environments. Hence the surrounding effect arises. And surrounding is the simplest gravitational model based on this effect. It means that with this corrected relativity, the gravitational mysteries of today simply do not exist. In other words, today the calculations of the gravitational predictions of relativity are wrong. And when this error is fixed then it remains no gravitational mysteries anymore.
How does this surrounding effect arise in relativity? Well, the main involved mechanism can be understood by a thought experiment. Let's imagine a sole wave propagating. Let's call this wave the "A" wave. And the universe is full of such waves, generated by the quasi relativistic quarks. If matter density of the universe is weak, each time A will encounter another wave (generated by a quark), it will not modify much the space-time deformation which is generated by A. But if matter density of the universe is strong, then there will be more of those encounters, and also the other waves encountering A will get a stronger energy. Those other waves will add also their strong contributions to the final space-time deformation. Therefore the relative contribution of A to this final deformation will be weaker. And finally the final space-time deformation generated by A will be weaker. This retrieves the surrounding effect.
How is it possible that such a miss occured in relativity for so long ?
"G is a variable" is a prediction of a chapter of relativity which has been completely missed for this long, indeed. This miss has been done for so long because special relativity hides it in a subtle way. The first degree of derivation of space-time structure has been relegated into the algebraic rule of Lorentz transforms. But it is much more than that! It is also a very important link between energy and space-time structure to be found in the first degree of derivation. So this chapter of relativity can be called "the first degree of derivation of space-time structure". Now it has to be understood, detailed and exploited. Some of its basic parts are worked in the above article. One of its result is that G is a variable following the rule of the surrounding effect.
The result is that the most important issues in todays physics are solved by the development of a new chapter in relativity. This chapter has been ignored for more than a century because it was hidden by its own simplicity. It is about the link between energy and space-time structure which uses the first degree of derivation of the metric. It does not contradict Einstein equation but shows that this equation must be executed with a variable G. After a small development of this new chapter, the constant value of G appears to be extremely restrictive and anthropocentric.
REMARKS
Deprecated versions of this study are still available on the web: Gravitational Model of the Three Elements Theory (GMTET), Gravitational Model of the Three elements Theory: Formalizing, Surrounding Matter Theory: first mathematical developments, Discrete relativity, Relativity in motion, Updated relativity. Please do not take them into account.
If Einstein equation is wrong, what is (or are) the new equations ?
Einstein equation is still correct, must be applied with a variable G. The fundamental equation of General Relativity is given by Lagrangian in vacuum. And this is the only fundamental one. From this Lagrangian equation in vacuum, taking into account equivalence between ad hoc energy distributions, an equation is constructed naturally, which yields the four-vectors describing locally space-time structure. I uses the four-vectors of the gravitational waves which are generated by the ad hoc equivalence energy distributions:
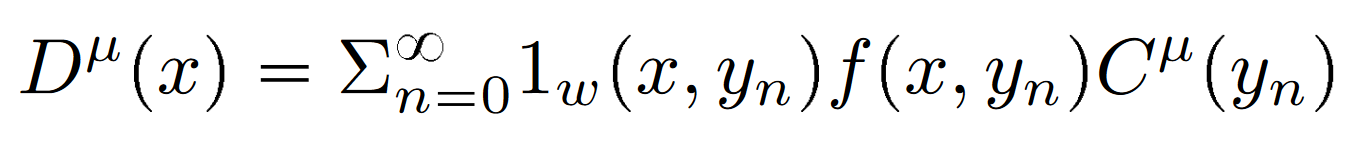 This equation follows the rule of the surrounding effect, which then arises in relativity. Also an equation of G can be given, which is a good approximation in most of the cases.
This equation follows the rule of the surrounding effect, which then arises in relativity. Also an equation of G can be given, which is a good approximation in most of the cases.
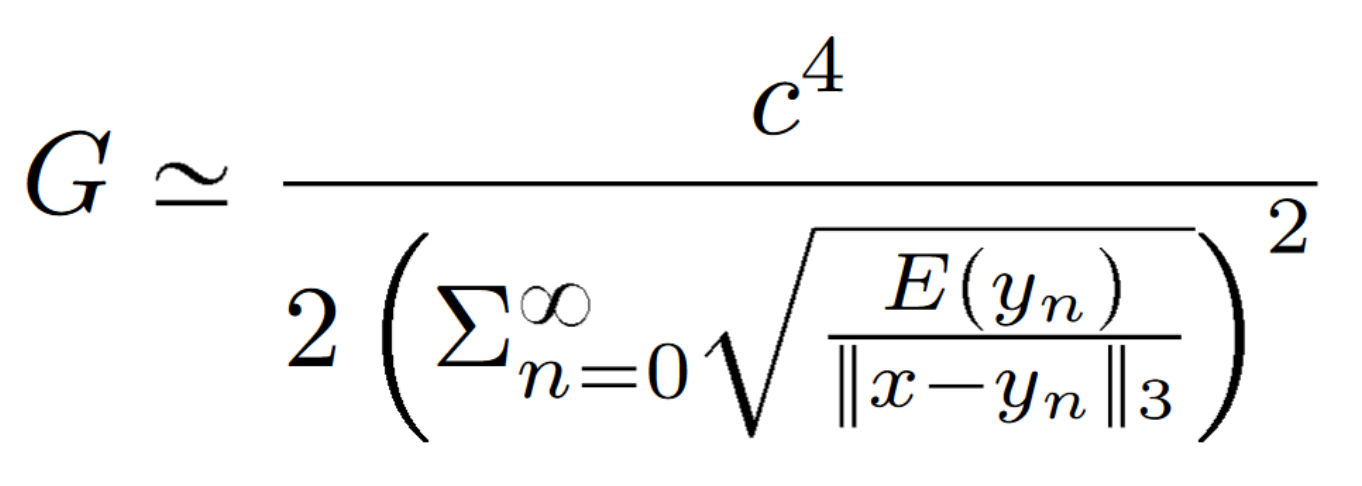
In this formulation it is only a (good) approximation. Its possible divergence is fixed the same way as the Olbers paradox is fixed. There is also a cutting-off value of 15 kpc for the interaction distance which is seen in the galaxy simulations. The square root over the energy of the relativistic or quasi-relativistic particles appears to be wired at first glance. But the so-called four-momentums of equation (1) giving rise to this equation of G are not four-momentums but simply four-vectors of contributions allowing to calculate space-time structure.
As a result, Einstein equation can still be used in most of the cases, that is, in the cases where the surrounding value is constant. But it must use the recalculated value of G given by this approximated equation. In more complicated cases, the only valid equation is conservation of Lagrangian in vacuum.
The singularities which are calculated by this new chapter of relativity are unbelieavable; the mistery of vacuum
With this new chapter, a mathematical demonstration shows that relativity predicts that there are singularities everywhere in space-time for a dimensionless particle alone in an empty universe. Of course this appears extremely wired, unbelievable and unrealistic. But this energy distribution is unrealistic in the first place. Indeed what is unrealistic is this thought experiment which tells you the story of an empty universe except for one and only particle ! Moreover, let's try to speak gently about this thought experiment, forgetting one minute the assumption of a constant G value.
This discussion is related to the lingering and old physics problems and questions about vacuum. It is already accepted that, in an empty universe, space-time structure can't be calculated, any possible structure would be valid since no determination exists and since the number of degrees of freedom are infinite for such a calculation. Now let's assume that one adds a dimensionless particle in such an empty universe. Then what happens ? Then any weak reaction of space-time to this new added particle would be weak, apart from the strongest possible one. It means that, yes, there must be a singularity everywhere. It is very much understandable that the whole space-time structure will bow, will creep and will lie down, watching the particle in an extremely docile and obedient way ! Moreover this singularity happens in a way which respect the spherical symmetry around the particle. These humble remarks, which are not mathematics, lead to the exact same result as the mathematical demonstration given by this new chapter of relativity. Of course the present discussion of the present paragraph here is not mathematics, it is not rigourous, it is only a humble thought. But it shows that the singularities which are calculated by this new chapter of relativity are understandable, even thought they appear wired and unbelievable at first glance. Moreover, they are much more prone to be the correct predictions of relativity than the usual one, for this energy distribution. Here usual relativity would predict a regular space-time structure, using solar system constant G value. By the way this would contradict Mach's principle (please refer to my article for this argument here about the Mach's principle).
Back to the problem of vacuum, now relativity shows that a surrounding effect is a central behavior of space-time. This derives also from this mathematical demonstration we were talking about above. With this effect, in vacuum an infinite elasticity of space-time is predicted. It means that any particle inserted into this empty universe would deform space-time in the strongest possible way. In vacuum every space-time structure is possible, and any added energy would see space-time reacting to it in the strongest possible way, elasticity is infinite. This new behaviour of vacuum gives the beginning of an answer to the old physics mystery of vacuum.
G constant measurements
From Cavendish to today, the measurements of this "constant" are extremely inaccurate and are even contradicting themselves.
This is a prediction of relativity. Even surrounding predicts different measured values of G depending of the astrophysical context.
In reality there might exist shielding mechanisms involved. It means that the denominator of the equation of G above must be corrected by insertion of an attenuation factor in the terms of the sum. There is a clue for that shielding to occur, with the existence of the two values of the "alpha" parameter of surrounding. Also there are clues to think that this phenomenon might be correlated to the Casimir effect. And this would not contradict the today's explanation of the Casimir effect, which is done with quantum mechanics.
For confirmation of this, a test is to measure G, successively, 2 times. For the first measurement the apparatus is located in the middle of a metal box. For the second measurement the box is taken out. If possible, metal walls are inserted between the attracted object and the attracting object, in a symmetrical way, that is, another similar wall is located on the opposite side with respect to the attracted object, at the same distance of the attracted object. The first measured value is predicted to be greater than the second one.
For the full understanding of this shielding effect, the following fractional formulation of equation (1) must be used, in place of the equation of G.
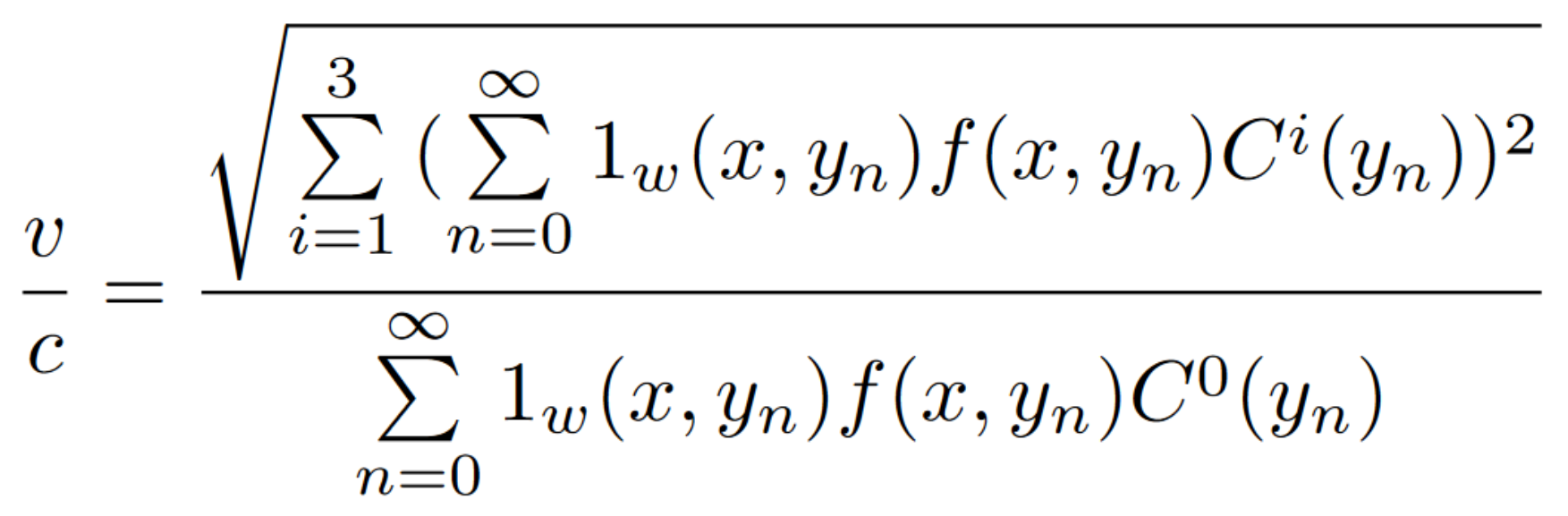 The first measurement decreases the denominator. The second one increases it. And if the two walls are inserted during this second measurement, it decreases the numerator.
The first measurement decreases the denominator. The second one increases it. And if the two walls are inserted during this second measurement, it decreases the numerator.
Looking forward for help
I need help for the realization of the G constant measurement (described above).
May 2024.
